Perturbing Material-Components on Stable Shapes
How Partial Differential Equations Fit into the Descriptions of Stable Physical Systems
Nonfiction, Science & Nature, Mathematics, Reference & Language, Reference, Education & Teaching| Author: | Martin Concoyle Ph.D. | ISBN: | 9781490723723 |
| Publisher: | Trafford Publishing | Publication: | January 16, 2014 |
| Imprint: | Trafford Publishing | Language: | English |
| Author: | Martin Concoyle Ph.D. |
| ISBN: | 9781490723723 |
| Publisher: | Trafford Publishing |
| Publication: | January 16, 2014 |
| Imprint: | Trafford Publishing |
| Language: | English |
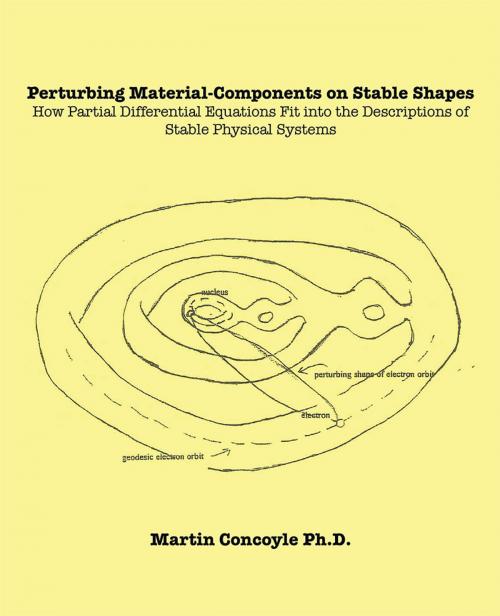
This book is an introduction to the simple math patterns that can be used to describe fundamental, stable spectral-orbital physical systems (represented as discrete hyperbolic shapes, i.e., hyperbolic space-forms), the containment set has many dimensions, and these dimensions possess macroscopic geometric properties (where hyperbolic metric-space subspaces are modeled to be discrete hyperbolic shapes). Thus, it is a description that transcends the idea of materialism (i.e., it is higher-dimensional so that the higher dimensions are not small), and it is a math context can also be used to model a life-form as a unified, high-dimension, geometric construct that generates its own energy and which has a natural structure for memory where this construct is made in relation to the main property of the description being, in fact, the spectral properties of both (1) material systems and of (2) the metric-spaces, which contain the material systems where material is simply a lower dimension metric-space and where both material-components and metric-spaces are in resonance with (and define) the containing space.
This book is an introduction to the simple math patterns that can be used to describe fundamental, stable spectral-orbital physical systems (represented as discrete hyperbolic shapes, i.e., hyperbolic space-forms), the containment set has many dimensions, and these dimensions possess macroscopic geometric properties (where hyperbolic metric-space subspaces are modeled to be discrete hyperbolic shapes). Thus, it is a description that transcends the idea of materialism (i.e., it is higher-dimensional so that the higher dimensions are not small), and it is a math context can also be used to model a life-form as a unified, high-dimension, geometric construct that generates its own energy and which has a natural structure for memory where this construct is made in relation to the main property of the description being, in fact, the spectral properties of both (1) material systems and of (2) the metric-spaces, which contain the material systems where material is simply a lower dimension metric-space and where both material-components and metric-spaces are in resonance with (and define) the containing space.